
So we learn in H.S.M.
Coxeter's wonderful Regular Polytopes (Dover, 1973).
When time permits, I may try to provide a systematic approach
to higher space. Dimensional analogy is an important tool,
when grappling the mysteries of hypercubes and their ilk. But
let's start at the beginning, and to simplify matters, and also
bring the focus to bear upon the most interesting ramifications
of the subject, let us concern ourselves mostly with regular
polytopes. You may wish to explore my links
to some rather interesting and wonderful polyhedra and polytopes
sites, at the bottom of this page. Check out an animated
GIF (108K) of an unusual rhombic spirallohedron.
The most symmetrical plane projection of
the four-dimensional star polytope {5/2,3,3}. This polytope is
bounded by 120 Great Stellated Dodecahedra, and has 600 vertices,
1200 edges, and 720 pentagrams.
Yes, we shall be speaking of the fourth dimension, and, well, the 17th dimension, or for that matter, the millionth dimension. We refer to Euclidean spaces, which are flat, not curved, although such a space may contain curved objects (like circles, spheres, or hyperspheres, which are not polytopes). We are free to adopt various schemes to coordinatize such a space, so that we can specify any point within the space; but let us rely upon Cartesian coordinates, in which a point in an n-space is defined by an n-tuplet of real numbers. These real numbers specify distances from the origins along n mutually perpendicular axes, such as the familiar x-axis and y-axis of the Cartesian plane.
In space of zero dimensions, the only figure possible is a point. It has no length, no breadth, no height. In space of one dimension, the only polytopes possible are line segments and points. A segment is said to be bounded by two points (its end-points). We may obtain a tessellation or close-packing of polytopes in this 1-space, by joining line segments point-to-point to fill the line of the 1-space.
In space of two dimensions (a plane), we may have points, segments, and polygons. Setting aside degenerate cases like the digon (a polygon with two coincident sides) and the apeirogon (a polygon with an infinite number of sides), the simplest possible polygon is the triangle. A triangle is bounded by three segments (its sides) and three vertices (the endpoints of its three sides, which fall into coincidence in pairs). Note that if the triangle be equilateral, it is also regular: all the sides have equal length, and all the interior angles are equal. However, if a polygon has more than three sides, to be equiangular is not to be regular: for instance, all rhombs are equilateral, but only that rhomb known as the square (or, two-dimensional measure polytope, or two-dimensional hypercube, or 2-cube) is equiangular, and regular.
A curious fact about regular polygons is that
some have Euclidean constructions, but most do not. That is, some
may be constructed with compass and unmarked straight-edge alone.
Among these are the equilateral triangle, the square, the pentagon,
the 17-gon, the 257-gon, and the 65,537-gon. It must be noted
at the outset that a regular polygon may be regular, yet not convex:
the sides may be equilateral and equiangular, yet cross one another,
forming star polygons. Some examples are given below, labeled
with their Schlafli symbols (Ludwig Schlafli was a pioneer
in the study of regular polytopes, and devised these symbols).
A regular convex n-gon has Schlafli symbol {n},
while a star n-gon in which every k-th vertex is
joined (of the n vertices of the "parent" n-gon,
taken cyclically) has Schlafli symbol {n/k}.
Three regular heptagons (7-gons), and a
regular star 24-gon.
Now, one thing which makes the study of regular
polytopes interesting is their symmetry; but another is their
departures from simple patterns and orderings. There are infinitely
many different regular polygons, but only nine regular polyhedra:
the famous five Platonic Solids, and the four less-known
Kepler-Poinsot polyhedra, which are stellations of the
Platonic pentagonal dodecahedron and icosahedron. In four dimensions,
there are sixteen regular polytopes: six convex, and ten starry;
but in each and every higher space, there are but three: the regular
simplex, the cross polytope or orthoplex, and the hypercube. These
are analogues of the Platonic Tetrahedron, Octahedron, and Cube.
The five Platonic Solids, so named because Plato described them in the Timaeus.
These are hidden-detail-removed projections:
look carefully at the polygonal outline of each projection, and
at the tiling of foreshortened polygons which compose that polygon.
For images of the Kepler-Poinsot star polyhedra, and of sections and projections of the regular star-polytopes in four dimensions, visit my star polytopes page. For a QuickTime movie of the solid sections of the regular star polytope {5/2,3,3}, go here.
Now: please observe that a polyhedron is bounded by polygons, which in turn are bounded by segments, which in turn are bounded by points. We say that a polyhedron is bounded by faces (polygons), edges (segments), and vertices (points). Note that every edge of a polyhedron is the join of two faces, and that every vertex of a polygon is the join if two sides. We have stumbled upon a kind of binary rule which applies to all simply-connected polytopes, I think: every (n-2)-dimensional bounding polytope is the join between exactly two (n-1)-dimensional bounding polytopes.
Thus in four dimensions, where 4-polytopes are bounded by 3-polytopes (polyhedra), every polygon is the join between exactly two polyhedra. Now, the geometry of four dimensions has always and rightly been regarded as fiendishly, demonically difficult. It is. However, one may gain a foothold in this exalted space, so often the very source and fount of fantastical magical powers and events, in the eyes of this or that supposed mystic, by carefully applying dimensional analogy.
Ludwig Schlafli, a Swiss, made his great advances in the study of higher space in the middle of the 19th century. His work went largely unnoticed, and in the 1880s others brought some of the same ideas forward, and even gained credit for what Schlafli had already achieved decades before. Oh, but there is a ponderous army of sines and cosines, of dot products and cross products, guarding the approaches to this magical realm. Stay, though! Not every pass is guarded; one can reach the unruly realm, using dimensional analogy!
In fact, one of the more significant contributors to the facts and theories of higher space was a person without any training in linear algebra or trigonometry. Her name was Alicia Boole Stott. While geometers in the great universities, a century past, were laboring upon the broad outlines of things polytopical, Alicia Boole Stott had already gone far beyond. Her principal tool was dimensional analogy.
Here is an application of dimensional analogy:
just as a hidden-detail-removed projection of a convex polyhedron,
onto a plane, induces a tiling of the projection's bounding polygon,
by one or more (usually smaller) polygons, so also a hidden-detail-removed
projection of a four-dimensional convex polytope, into a 3-space,
induces a tiling of the projection's bounding polyhedron, by one
or more (usually smaller) polyhedra.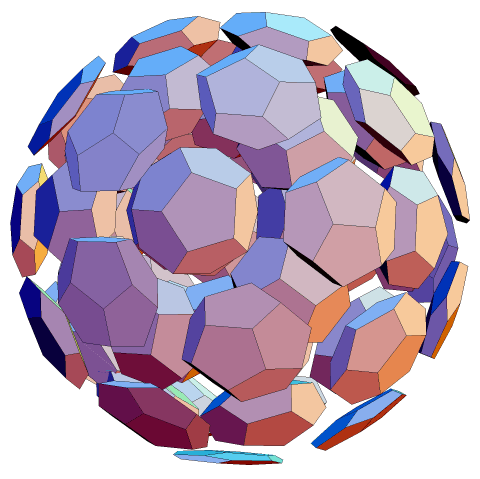
A pentagonal-dodecahedron-first, hidden-detail-removed
projection of the regular 4-polytope {5,3,3} (or 120-cell), into
a 3-space. The variously foreshortened dodecahedral cells have
been exploded apart slightly so that some of the inner dodecahedra
are visible. Note that, from the 4-space, all the dodecahedra
would be visible at once, exploded or not.
These hidden-detail-removed projections of 4-polytopes into a 3-space were something I devised using the software, Mathematica. See my Mathematica page, or just download the Regular Polytopes notebook.
It is quite significant that such projections induce a tiling of polyhedra, composing a larger polyhedron. The subject here becomes rather vast and also dense, and relates to my zonotopal completions of convex polytopes, and to quasicrystals and zonotiles, and to the stellations of convex polytopes. I hope to describe these ideas in more detail soon. Go here for three animated GIFs which compare the processes of completion, stellation, and faceting, with a short discussion.
From any convex polytope we may obtain related
figures, the so-called rectified and truncated forms. A rectified
polytope has for vertices the mid-edge points of its parent, while
a truncated regular polytope is typically imagined to be truncated
by hyperplanes perpendicular to the vectors to its vertices, to
just such a depth as would create a regular 2n-gon, from
any one of the bounding n-gons. For instance, the rectified
cube (or octahedron) is the cuboctahedron, bounded by six squares
and eight triangles, while the truncated cube is bounded by six
octagons and eight triangles. In space of four dimensions, the
rectified 120-cell, or r{5,3,3} is bounded by 120 Archimedean
icosidodecahedra, and 600 Platonic tetrahedra.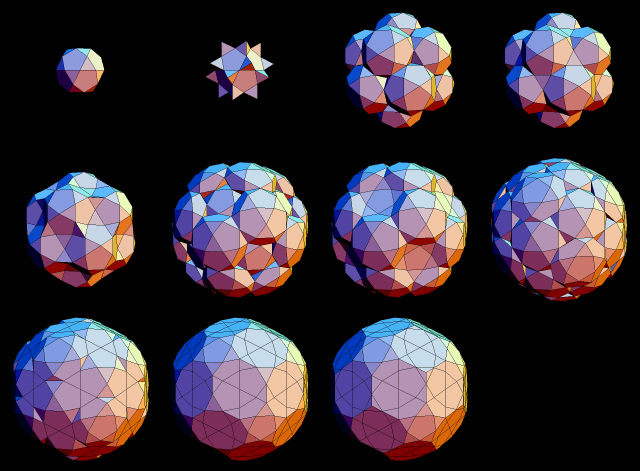
Above: how the hidden-detail removed, icosidodecahedron-first
projection of the rectified 120-cell is built up, from its central
icosidodecahedron, to its bounding polyhedron (from left to right,
and top to bottom).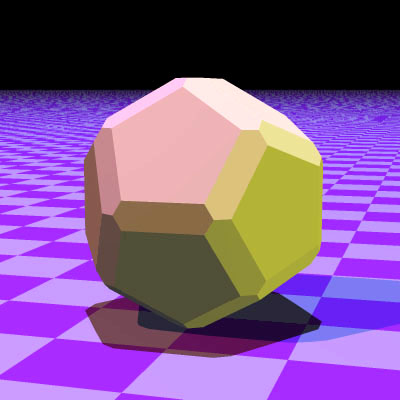
Above: truncation of a polytope need not be of the type defined above. Here, a Platonic pentagonal dodecahedron has been slightly truncated edge-wise.

An extract from my favorite chapter of Coxeter's
Regular Polytopes, in which he begins the discussion of
the projection of hypercubes (called measure polytopes, if of
unit edge length, for then they define the unit of n-dimensional
content) into zonotopes, and the fascinating subject of eutactic
stars. Of which, more here!
Here is another example of dimensional analogy:
Given a close-packing of equal cubes, filling a region of 3-space, we can take their sections by a plane. The result is a close-packing of polygons which fill a region of the sectioning plane. Now, let the cubes be exploded apart slightly, and take a section by a plane perpendicular to the line joining opposite vertices of any one cube. Depending upon the position of this sectioning plane, the polygons change shape. Here is one such section:
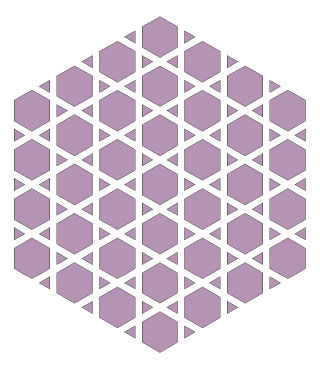
Section of an array of 3-cubes.
Among the regular space-fillings in four dimensions is that of the 24-cell, {3,4,3}, which is bounded by 24 Platonic octahedra. Fill a region in the 4-space with {3,4,3}'s, exploded apart slightly, and take their section by a 3-space perpendicular to the line joining opposite octahedra, of any one {3,4,3}. Here is one such section:
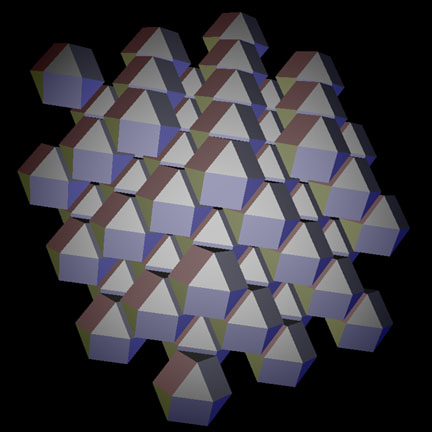
By dimensional analogy, just as the plane section of close-packing polyhedra gives close-packing polygons, so also the solid section of a close-packing of 4-polytopes gives close-packing polyhedra. In this case, the close-packing is semi-regular, with Archimedean cuboctahedra and Platonic octahedra.
Links to polyhedra and polytopes:
George Hart's Pavilion of Polyhedrality
George Olshevsky's Four-dimensional polytopes pages
Wolfram Research's Polytope Pages!
Mark Newbold's polyhedra and polytopes (Java applets, stereo pairs)
David Eppstein's Totally Awesome Geometry Junkyard
Flatland: A Romance of Many Dimensions
James R. Buddenhagen's Uniform Polyhedra
Jim McNeill's Great Polyhedra and Tessellation Site